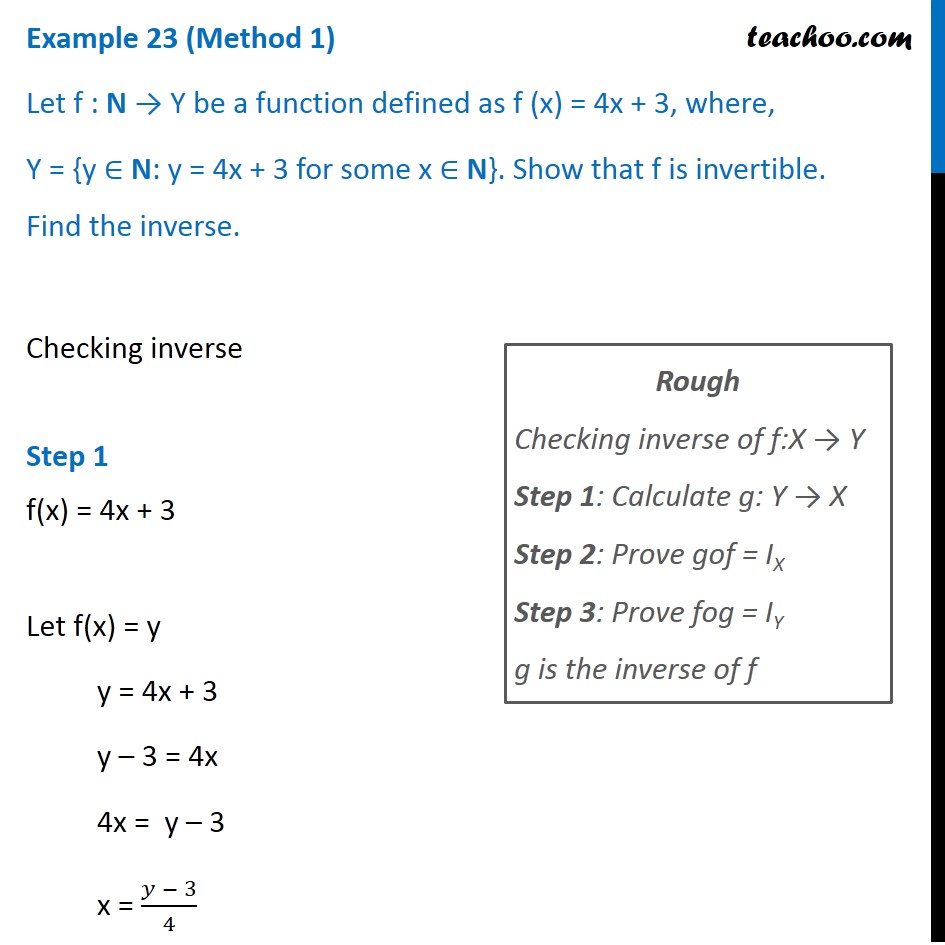
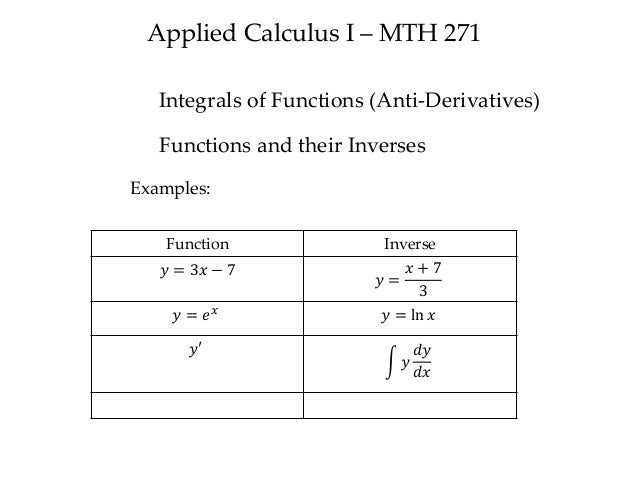
Jun 15, · What is an inverse function?Dec 07, 10 · Find the inverse of the function f(x) = e^(3x 5) 5 Educator answers Math Latest answer posted November 29, 10 at AM find the inverse formula for the function f(x)= 3x7 4 EducatorWe have the function f of X is equal to X minus 1 squared minus 2 and they've constrained the domain to X being less than or equal to 1 so we have the left half of a parabola right here and they constraint it so that it's not a full u parabola and I'll let you think about why it would make that would make finding the inverse difficult but let's try to find the inverse here and a good place to
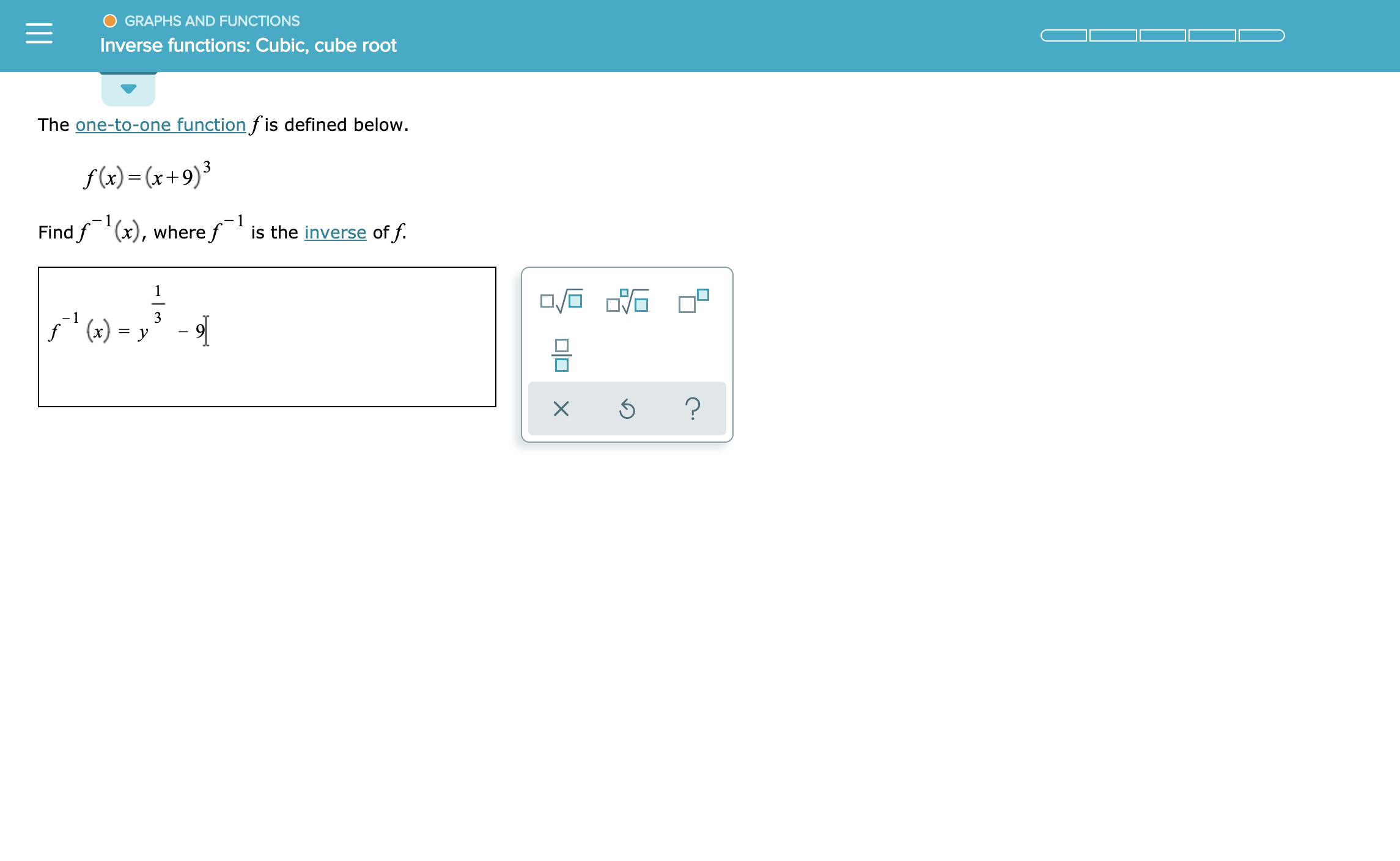
Answered Graphs And Functions Inverse Functions Bartleby
Find the inverse of the function y=-3/x+4
Find the inverse of the function y=-3/x+4-New questions in Mathematics 4 pts u Question 14 Determine all the possible values of x if ALMN has the following side lengths LM = 6x 3, MN = 4x 17, LN = x 40Mar 13, 12 · y/3 = x^2 x = √(y/3) Similar Questions math please help use an inverse trig function to write thetdjacent side of theta is labeled(x 1) The


Examview A 44 Inverseoffunctions2 Tst
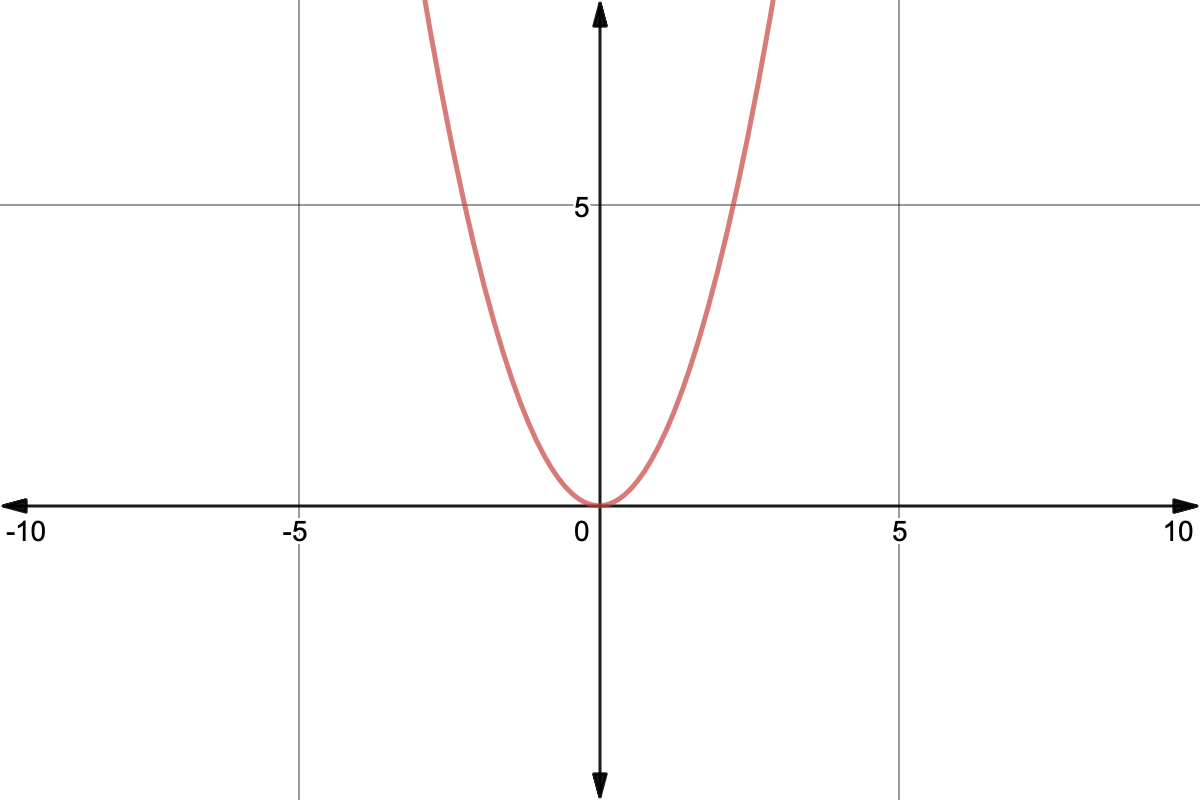
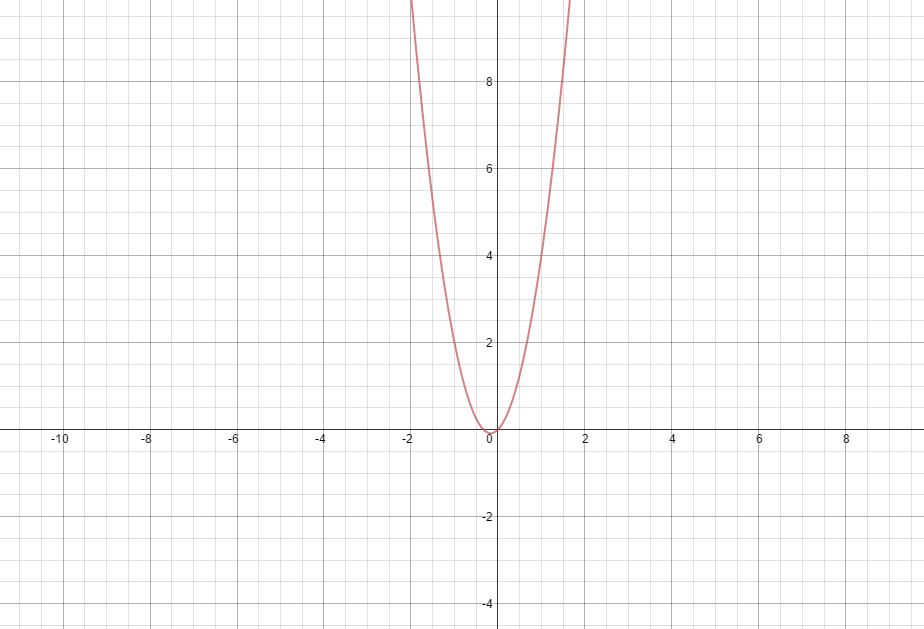
Inverse functions In Example 1, you can use the equation solved for x to write the inverse of f by switching the roles of x and y f(x) = 2x 3 original function g(x) = x − 3 — 2 inverse function Because inverse functions interchange the input and output values of the original function, the domain and range are also interchangedFeb 21, 11 · let's think about what functions really do and then we'll think about the idea of an inverse of a function so let's start with a pretty straightforward function let's say I have f of X is equal to 2x plus 4 and so if I take F of 2 f of 2 is going to be equal to 2 times 2 plus 4 which is 4 plus 4 which is 8 I can take F of 3 F of 3 which is 2 times 3 plus 4 which is equal to 10 all right 6 plusExample 2 Find the inverse function of f\left( x \right) = {x^2} 2,\,\,x \ge 0, if it existsState its domain and range This same quadratic function, as seen in Example 1, has a restriction on its domain which is x \ge 0After plotting the function in xyaxis, I can see that the graph is a parabola cut in half for all x values equal to or greater than zero
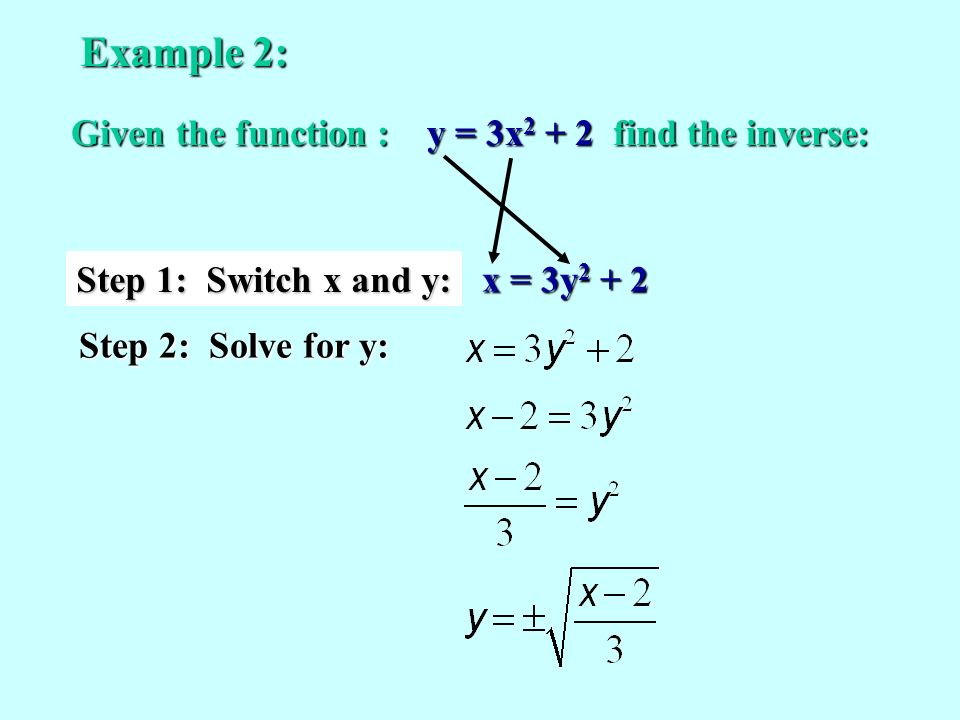
What is the inverse function of y=x^3 x Saman, First of all I know that y = x 3 x has an inverse since it is an increasing function I know this because the sign of the derivative is positive for every real number x My guess is that this comes from a calculus problem here you are to find the derivative of the inverse at some pointLet mathf(x) = x^3 x/math I'm assuming that mathf\mathbb{R}\to \mathbb{R} /math Since mathf(x)/math is a bijection mapping (the proof is very simple and I hope you know that) Therefore its inverse exists uniquely For finding theAlgebra College Algebra (MindTap Course List) Find the inverse of the function defined by each equation y = 3 x 2 more_vert Find the inverse of the function defined by each equation y = 3
Free functions inverse calculator find functions inverse stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie PolicyFree math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutorWhat is the inverse of the function?
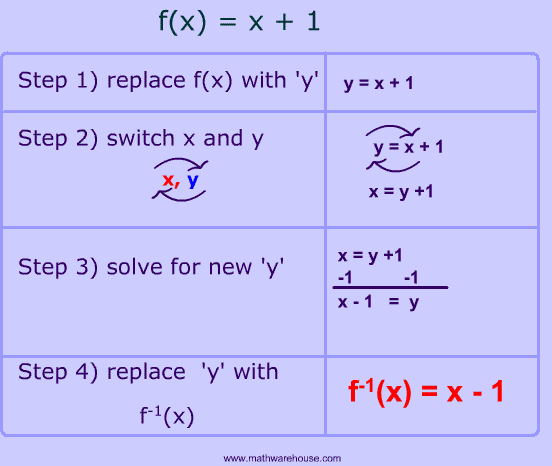


Inverse Of A Function In Math Tutorial Explaining Inverses Step By Step Several Practice Problems Plus A Free Worksheet With Answer Key


Derivatives Of Inverse Functions
Dec 05, 19 · Function pairs that exhibit this behavior are called inverse functions Before formally defining inverse functions and the notation that we're going to use for them we need to get a definition out of the way A function is called onetoone if no two values of \(x\) produce the same \(y\) Mathematically this is the same as saying,And then find the possible values for y If you obtain two values of y, then the inverse of f(x) is not a functionFind the inverse, and rename the resulting "y" as "f –1 (x)"It's usually easier to work with "y"



What Is The Inverse Function Of Y X 3 Quora
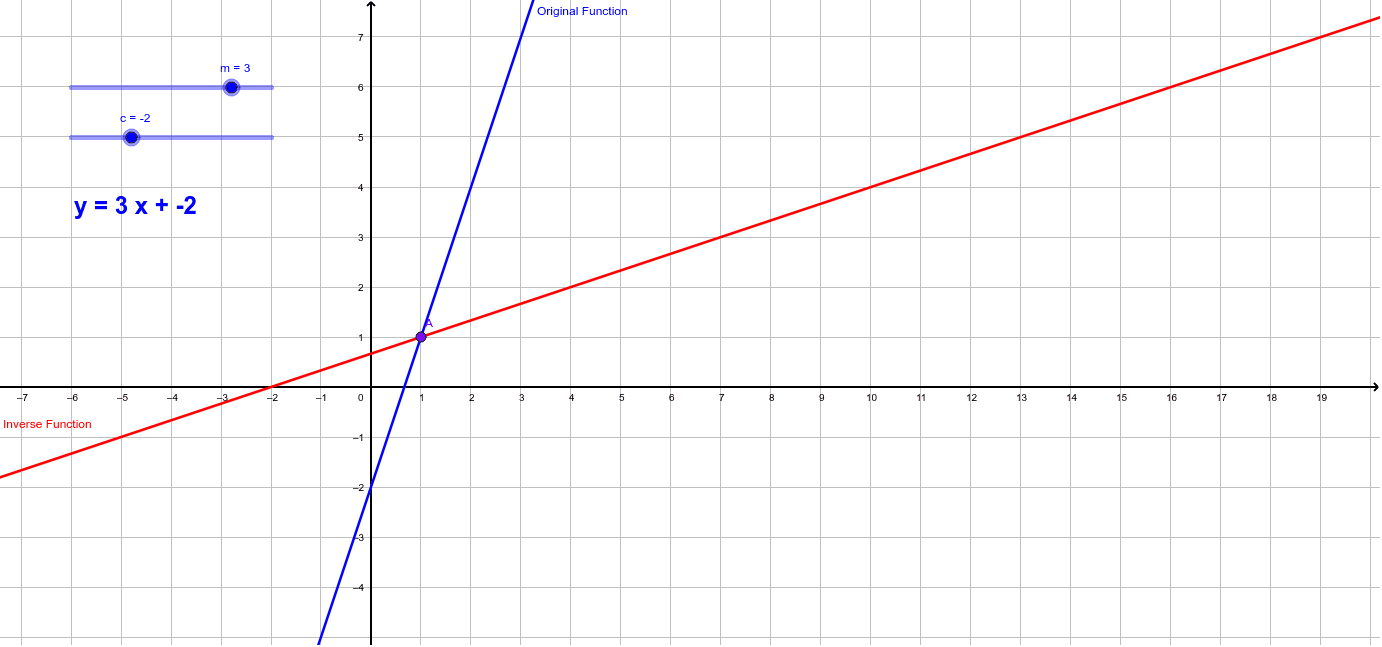


Linear Functions And Their Inverses Geogebra
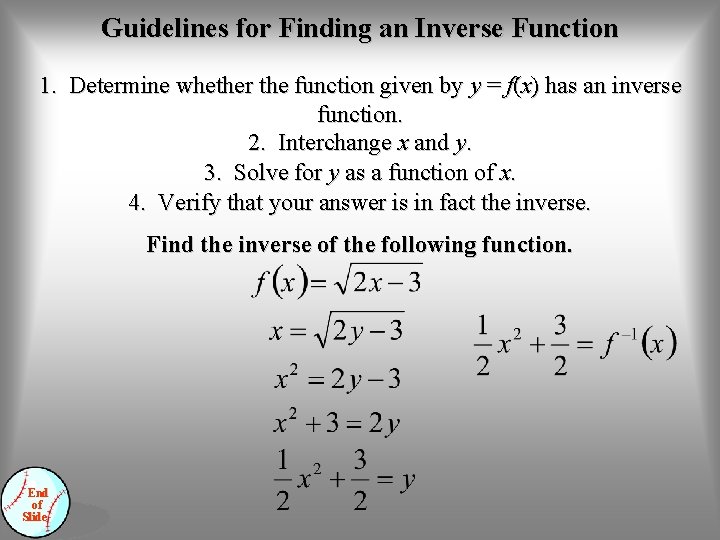
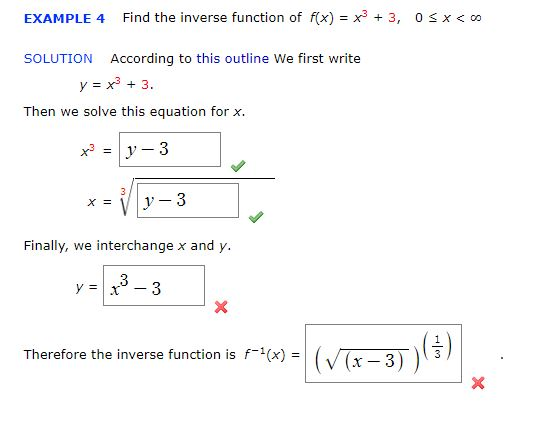
In this case, while there is an explicit formula for the inverse, it is complicated Sometimes, there just is no way to write down the function explicitly in terms of a formula But it is already instructive to ask why the function has an inverse A function has an inverse if and only if it is one to one and onto (that is, a bijection)Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutorJun 02, 18 · This is one of the more common mistakes that students make when first studying inverse functions The process for finding the inverse of a function is a fairly simple one although there is a couple of steps that can on occasion be somewhat messy Here is the process Finding the Inverse of a Function



Derivatives Of Inverse Functions



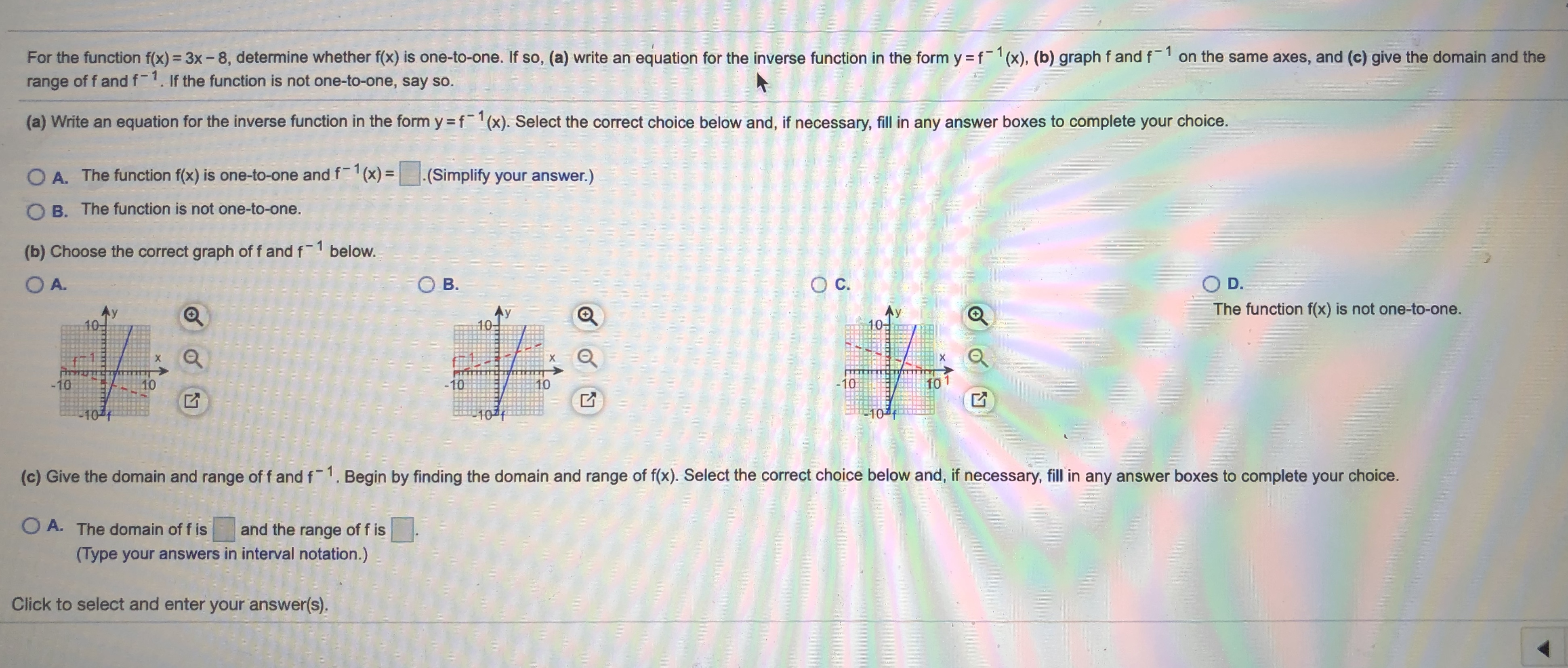
Examples Of Math Problems Find The Inverse F X 3x 8 4x 5
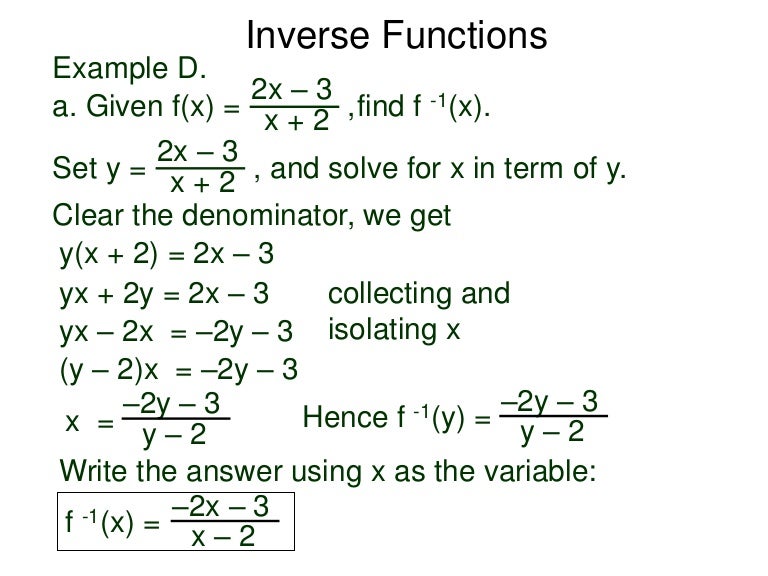
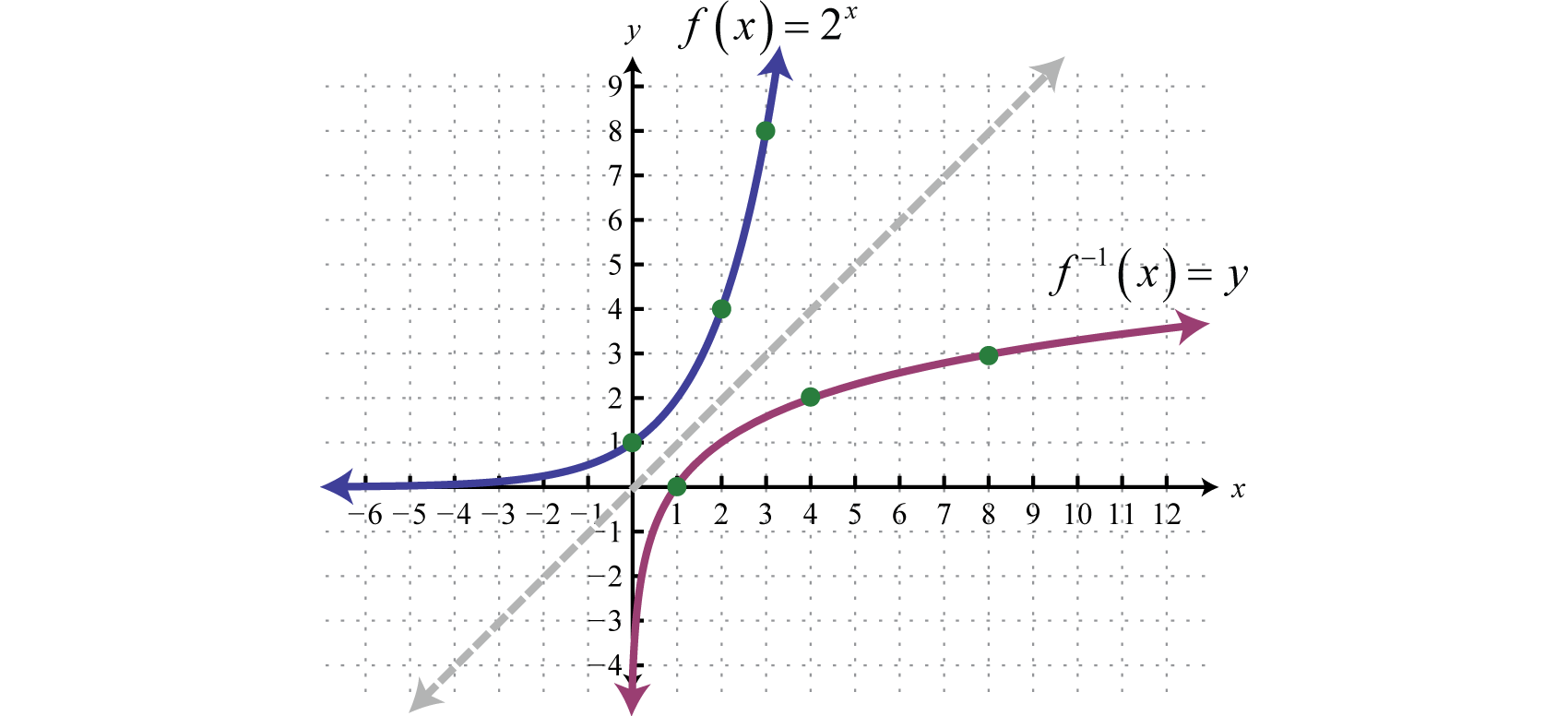
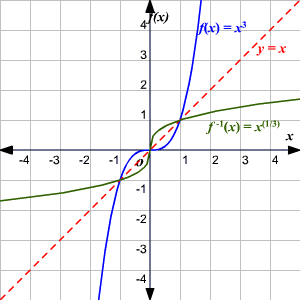
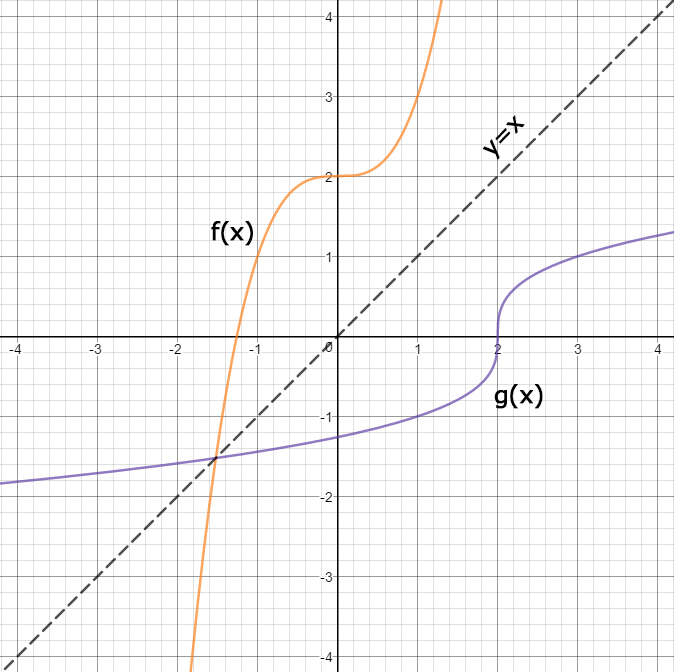
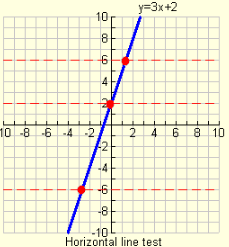
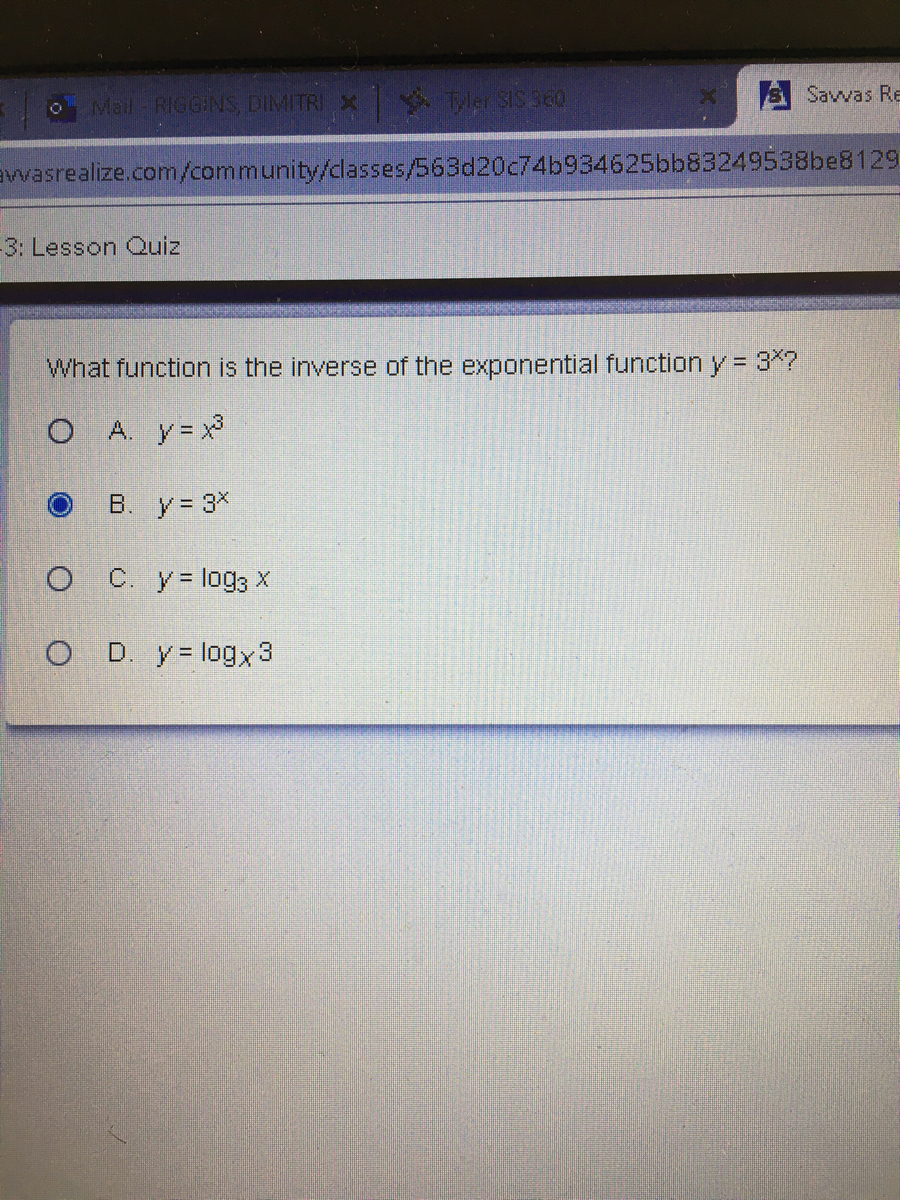
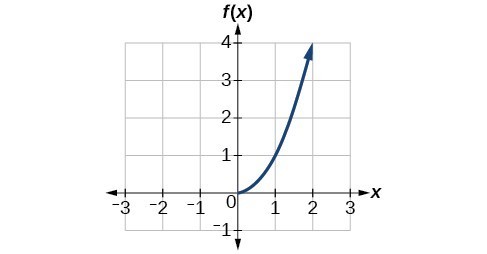
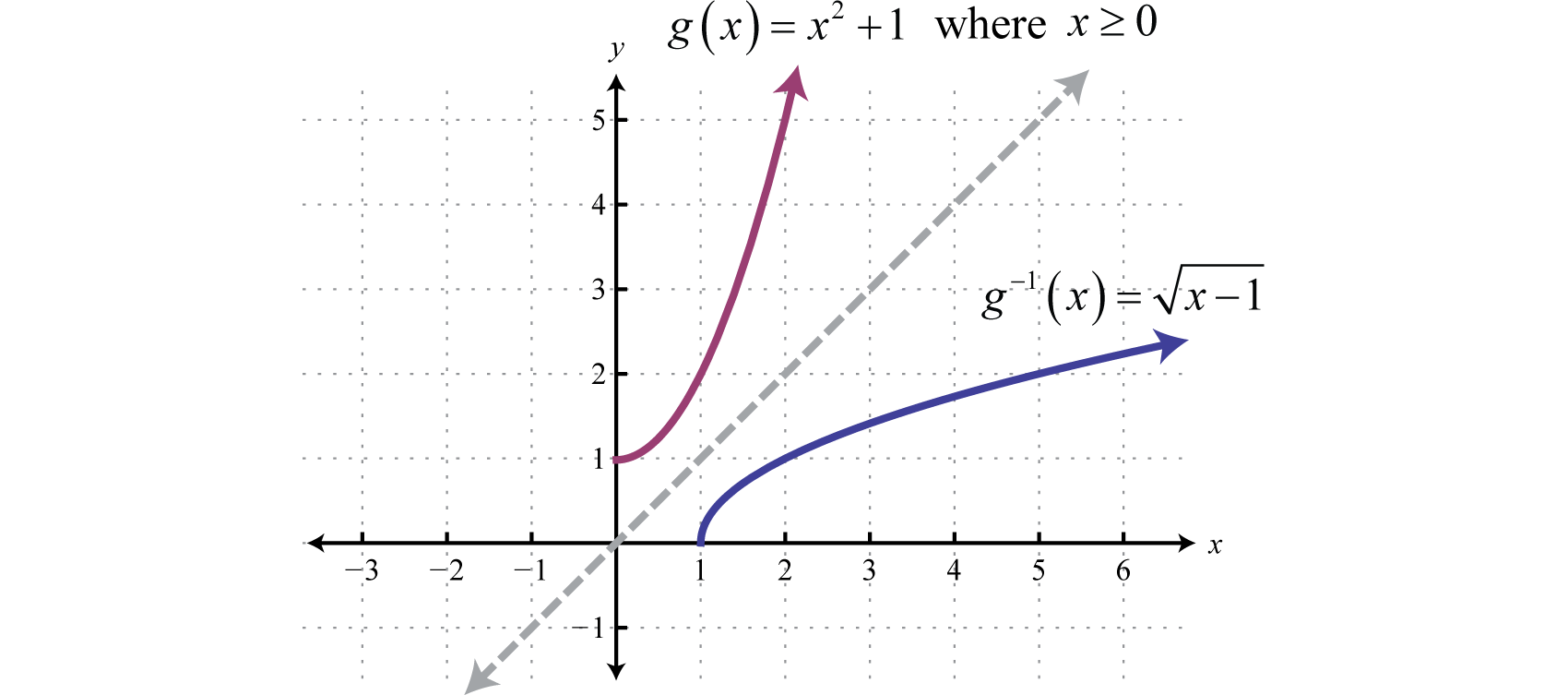
Aug 16, 01 · In mathematics, an inverse function (or antifunction) is a function that "reverses" another function if the function f applied to an input x gives a result of y, then applying its inverse function g to y gives the result x, ie, g(y) = x if and only if f(x) = y The inverse function of f is also denoted as As an example, consider the realvalued function of a real variable given by f(xThe restricted function passes the Horizontal Line Test, so the inverse will now be a function Since the domain of the original function is –2 < x < 0 and the range is –2 < y < 0, then the domain of the inverse will be –2 < x < 0 and the range will be –2 6 4 Use Inverse Functions Recall From Chapter Derivatives Of Inverse Functions From Equation Video Khan Academy Then the inverse is y = sqrt(x – 1), x > 1, and the inverse is also a function If you've studied function notation, you may be starting with "f(x)" instead of "y"In that case, start the inversion process by renaming f(x) as "y";Show all of your work for full credit Inverse of the given function, y=sqrt 9x And, its domain is, (infinity, 9 Find the inverse of the linear function y=1/2x3 f(x)= 2x6Jul 05, 18 · The answer is b y = 3(x5) To find inverse functions Swap x and y x = 1/3y 5 Solve for y y = 3(x5) Thank you so much! 4 1 Inverse Functions T Ppt The Function E X And Its Inverse Lnx Powerpoint Presentation Free Download Id Nov 29, 15 · How do you find the inverse of #y = 3^x#?Precalculus Functions Defined and Notation Function Composition 1 Answer Özgür Özer Nov 30, 15 #f^1=log_3x# Explanation #y=3^x# #=>log_3y=log_3(3^x)# (logarithms of both side are equal) #=>x=log_3y# #=>f^1=log_3x# Answer linkDefinition of Inverse Function Before defining the inverse of a function we need to have the right mental image of function Consider the function f(x) = 2x 1 We know how to evaluate f at 3, f(3) = 2*3 1 = 7 In this section it helps to think of f as transforming a 3 into a Logarithmic Functions And Their Graphs Solved 4 A Complete The Table And Graph The Function Y Chegg Com Here's a general procedure for finding inverses If the equation uses function notation, replace the "f(x)" with a "y"The inverse of a function can be found geometrically by reflecting the graph of the function over the line \(y=x\text{}\) Figure 039 The lefthand plot shows that after reflecting \(f(x)=x^2\) across \(y=x\) the result is not a function The righthand plot shows that under aJan 29, 15 · Myra uses an inverse variation function to model the data for the ordered pairs below (2, 30), (3, ), (4, 15), (5, 12), (6, 10) Which statement best explains whether an inverse variation function is the best model for the data? Solved 10 What Is The Inverse Function Of F X 11 Chegg Com Finding Inverse Functions Linear Video Khan Academy What is the domain of the inverse?The calculator will find the inverse of the given function, with steps shown If the function is onetoone, there will be a unique inverseThe function g(x) sends a value 'x' to 'y' The inverse function just brings 'y' back to 'x' So all you need to find is the value of 'x' that makes You can either guess and check, use a table, or a calculator In either method, you'll find that This means that (just apply the inverse to both sides of the last equation) Math Scene Functions 2 Lesson 6 Inverse Functions Inverse Of Square Root Function Chilimath Question find the inverse of the following function y=(2^x)3 Answer by jsmallt9(3758) (Show Source) You can put this solution on YOUR website!Let y = 3 x − 2 Interchanging x and y x = 3 y − 2 Now Solving for y Therefore the inverse function is Q Giv en find hThe inverse is usually shown by putting a little "1" after the function name, like this f1 (y) We say "f inverse of y" So, the inverse of f(x) = 2x3 is written f1 (y) = (y3)/2 (I also used y instead of x to show that we are using a different value) Back to Where We Started The cool thing about the inverse is that it should give us back What Is The Inverse Function Of Y 3x 4 2 Socratic Inverse Functions Finding the Inverse of a Polynomial Function Two functions f f and g g are inverse functions if for every coordinate pair in f, (a, b), f, (a, b), there exists a corresponding coordinate pair in the inverse function, g, (b, a) g, (b, a) In other words, the coordinate pairs of the inverse functions have the input and output interchangedSolved Find the inverse of the function y=3^x By signing up, you'll get thousands of stepbystep solutions to your homework questions You canStatistics You have an SRS of 15 observations from a Normally distributed population Finding The Inverse Function Algebraically Expii Use The Graph Of A Function To Graph Its Inverse College Algebra Mar 26, 18 · OK, so I want to find the inverse of the function y=3xe^x I started out by rewriting the equation as x=3 y e^y, solve for y Then ln(x)=ln(3) ln(y) y(ln(e)) ln(x)=ln(3) ln(y) y And here's where I get stuck I tried isolating "y" ln(y) y = ln(x)ln(3) But where do I goThe parent function is y 3 x which is the inverse of y x3 To graph y 3 x, start with the graph of y x3 Reflect the graph over the line y x To graph y 2 3 x 7, translate the reflected graph 7 units to the right and 2 units up To find the inverse of a function, you use an inverse process to solve for y after switching variablesInverse function of the given function is another function that do exactly opposite things To find the inverse function, first, we rewrite the function in the form of {eq}x=f(y) {/eq} and solve Solution Find The Inverse Of The Function F X X 1 3 2 I Am So Confused Can Someone Please Help Me Fr A X 2 3 B X 1 3 2 C Y 2 3 D Doesn 39 T Exist Find The Inverse Of The Function Y 3 X 4 Brainly Com Ch33H8152tex 11/7/06 12 46 Page 332 Module 7 Inverse Trigonometric & Hyperbolic Functions A Inverse Functions If y=3x −2, then by transposition, x = y2 3 The function x = y2 3 is called the inverse function of y = 3x − 2 Inverse trigonometric functions are denoted by prefixingthefunction with 'arc' or,more comNov 22, 13 · how do you expand f(x)=4x^32x in order to solve for the inverse of f(x) asked Mar 5, 14 in ALGEBRA 2 by rockstar Apprentice inversefunction;May 08, 08 · If you are asking for how to evaluate the inverse of f(x) at x=1, then you want to start with the inverse relationship, x= y^3 y 4 I assume you still meant that the original function was a cubic, and substitute 1=x; Functions Inverses Ppt Download A For A Function To Have An Inverse It Must Be So Which One Of The Following Function Has An Inverse F X X 2 Jan 29, 09 · The question only asks about one particular point, so it's pointless to find out what the entire inverse function is (Assuming that, since the question asks about an inverse, the inverse exists) Share Share Related Threads on How to find the Inverse of f(x) = 3x(e^x) Inverse of y = (e^x)3/(e^x)1 Last Post;The function y = log b x is the inverse function of the exponential function y = b x Consider the function y = 3 x It can be graphed as The graph of inverse function of any function is the reflection of the graph of the function about the line y = xStack Exchange network consists of 176 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack Exchange Find The Inverse Function Of Y 3x Brainly In Horizontal Line Test For Function To Have Inverse Expii 141 Determine the conditions for when a function has an inverse 142 Use the horizontal line test to recognize when a function is onetoone 143 Find the inverse of a given function 144 Draw the graph of an inverse function 145 Evaluate inverse trigonometric functionsIn mathematics, an inverse function is a function that undoes the action of another function For example, addition and multiplication are the inverse of subtraction and division, respectively The inverse of a function can be viewed as reflecting the original function over the line y = x Solved 3 Determine A Formula For The Inverse Function For Chegg Com Review Relation A Mapping Of Input Values X Values Onto Output Values Y Values Here Are 3 Ways To Show The Same Relation Y X 2 X Y Ppt Download How To Find Domain And Range Of The Inverse Of A Relation Sat Math Graphing Logarithmic Functions Inverse Functions Made Easy How Do You Find The Inverse Of Y Log 3x 2 Socratic Solution Find The Inverse Of Each Function Is The Inverse A Function F X 3x 2 F X X 2 5 Inverse Of Rational Function F X 2 X 3 Youtube Answered For The Function F X 3x 8 Bartleby Inverse Functions Definition A Function Is A Set Of Ordered Pairs With No Two First Elements Alike F X X Y 3 2 1 4 7 6 9 12 Ppt Download Derivatives Of Inverse Functions Summary Functions Siyavula Solved Find The Inverse Of The Function F X X 2 3x Chegg Com Ex Find The Inverse Of A Square Root Function With Domain And Range Youtube Answered Graphs And Functions Inverse Functions Bartleby Examview A 44 Inverseoffunctions2 Tst Answer In Algebra For Vidhi 3 7 Derivatives Of Inverse Functions Mathematics Libretexts Inverse Functions Example 23 Let F X 4x 3 Where F N Y Show F Is Invertible Inverse Functions Find Inverse Function Verbally Graphically Algebraically Problems Answers Solved Worksheet On Inverse Of Function Mcr3u Youtube Derivatives Of Inverse Functions Geneseo Math 222 01 Functions What Is The Domain And Range For The Following Function And Its Inverse F X 3x 1 2 Mathskey Com Solution Find The Inverse Of Each Function Then Graph The Function And Its Inverse 9 Y 3x 2 Finding Inverse Functions Radical Video Khan Academy Finding The Inverse Of A Function Youtube Finding The Inverse Function Algebraically Expii Graphically Represent The Inverse Of A Function A Plus Topper 1 Find The Inverse Of The Function Y 3x Ppt Download Functions Inverses Ppt Download What Is The Inverse Function Of F X 5x 3 4x 2 3x 4 Socratic Y 3x 2 Inverse 12x1 05 01 Inverse Functions 11 Question Video Finding The Inverse Of A Function Containing An Exponential Function Nagwa Finding Inverse Functions Quadratic Example 2 Video Khan Academy Inverse Of Square Root Function Chilimath Solved 3 10 Find The Inverse Of The Function F X For T Chegg Com Solved Find The Inverse Of The Function Y 3x 4 Is The In Chegg Com Work Out The Inverse Function For Each Equation A Y 2 X 5 B Y 4 X 7 C Y X 2 1 D Y X 4 3 Example 1 Find An Inverse Relation Find An Equation For The Inverse Of The Relation Y 3x 5 Write Original Relation Y 3x 5 Switch X And Y X Ppt Download 41 Find The Inverse Function For F X 3 X 7 Youtube Solved Find The Inverse Function Y E Quberoot X Select Chegg Com What Is The Inverse Function Of Y X 3 Quora How Do You Find The Inverse Of Y 3x 2 X And Is It A Function Socratic Answered 8 F X For The Function Y 3 X2 A Bartleby Inverse Of Derivative Function The Integral October 28 09 5 3 Inverse Functions Objectives Ppt Functions Inverse Of A Function Powerpoint Presentation Free Download Id Finding Inverse Functions Linear Video Khan Academy What Is The Inverse Of 8x 3 3x Quora Answered What Function Is The Inverse Of The Bartleby Use The Graph Of A Function To Graph Its Inverse College Algebra Kak Najti Obratnuyu Funkciyu 4 Shagov S Illyustraciyami Composition And Inverse Functions Solved Find The Inverse Function Of F X X3 3 0 X Chegg Com Composition And Inverse Of Functions Solved Find The Inverse Function Of Y 1 X 3 X 1 X Chegg Com Logarithmic Functions And Their Graphs 11 4 Inverse Relations And Functions Ppt Download 3 Ways To Find The Inverse Of A Quadratic Function Wikihow 8 Find The Inverse Of The Function Y 3 X 4 Brainly Com Inverse Functions Ppt Download Module 6 Review Inverses Table Of Contents Ppt Download How To Find Inverse Of An Exponential Function Y 3e 3x Youtube Ex 1 Find The Inverse Of A Function Youtube Inverse Functions Section 3 3 Set X Set Y Remember We Talked About Functions Taking A Set X And Mapping Into A Set Y An Inverse Ppt Download If Y F X X 3 X 5 And G Is The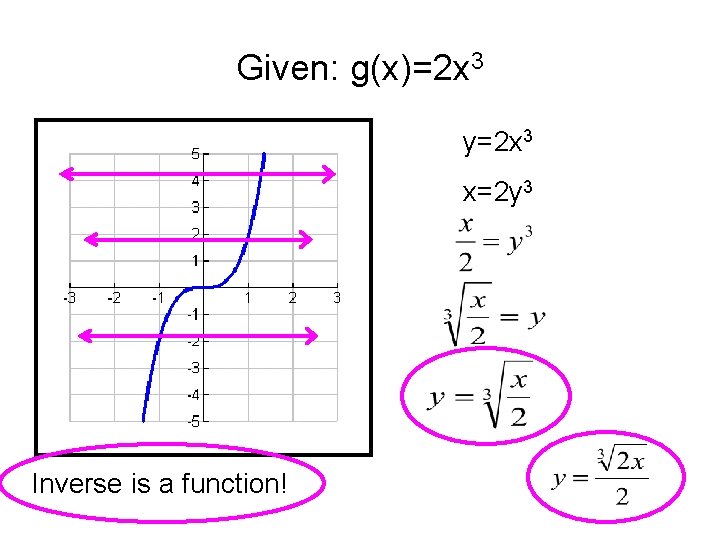






















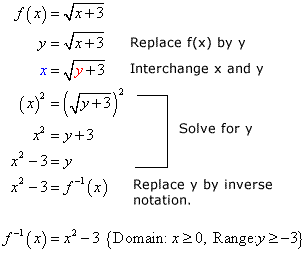


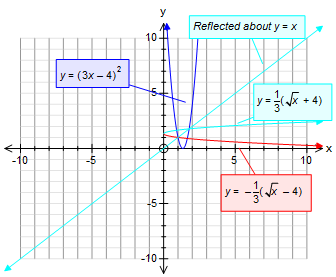


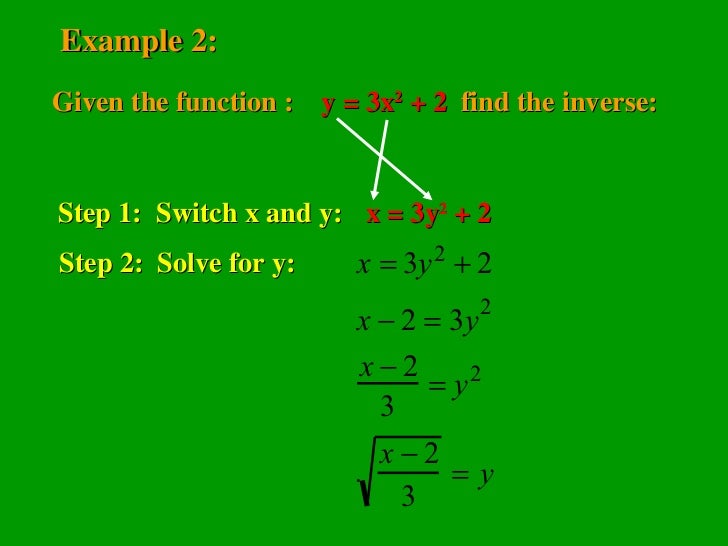





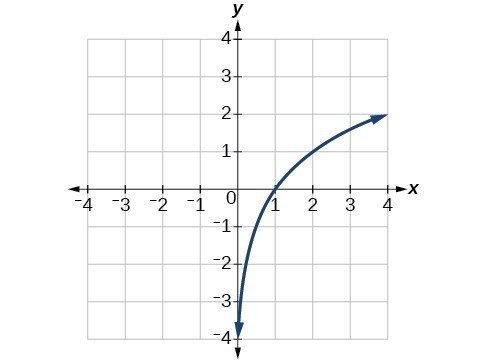


























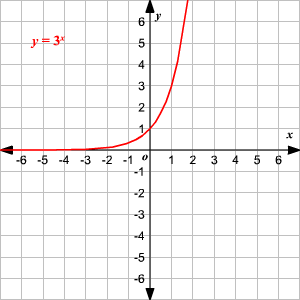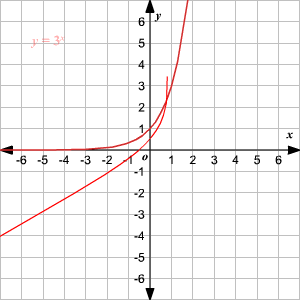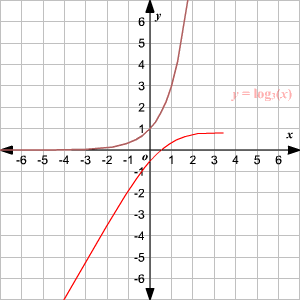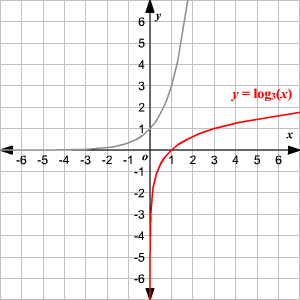





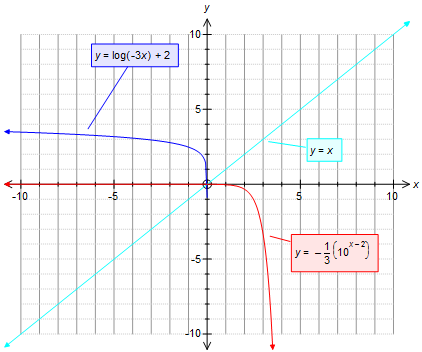


















































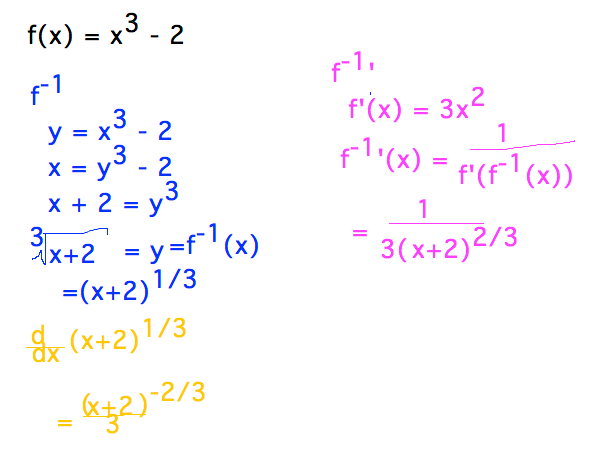






















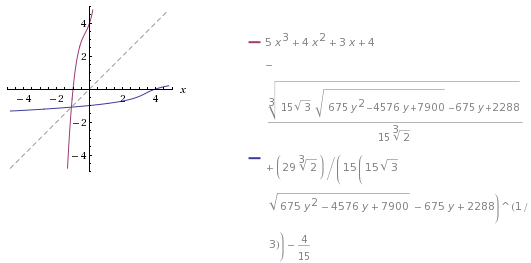





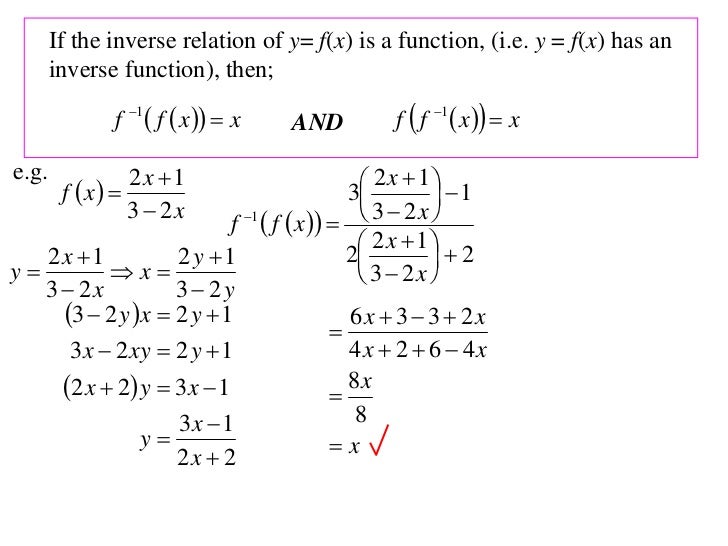


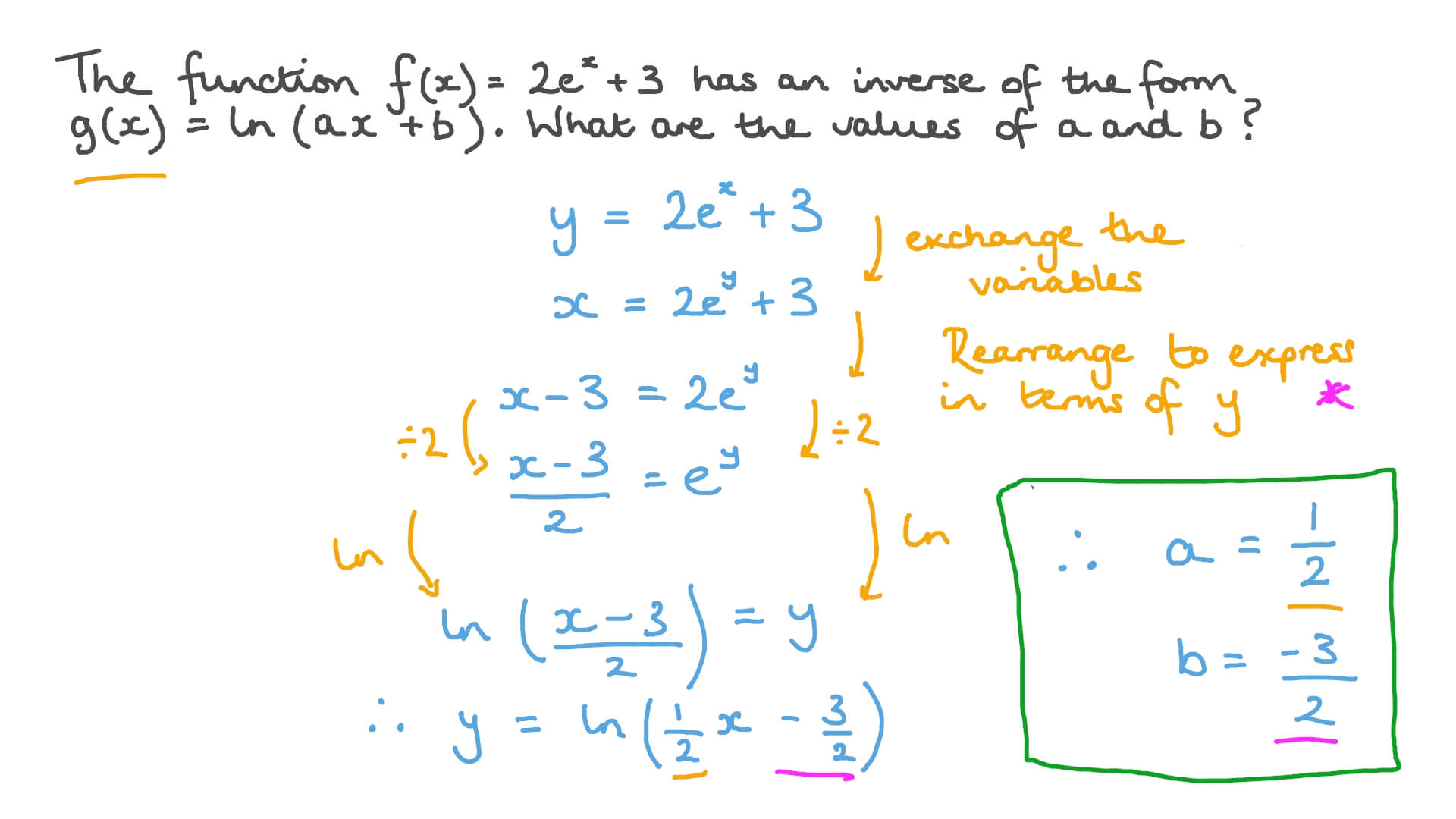





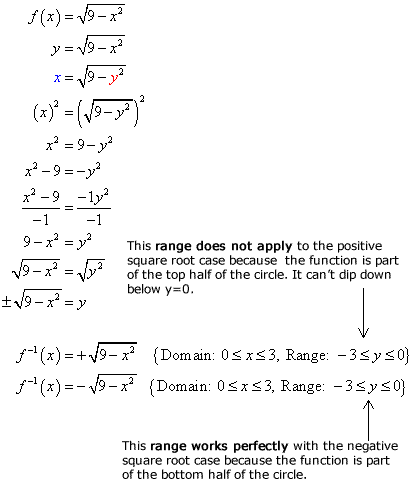

















































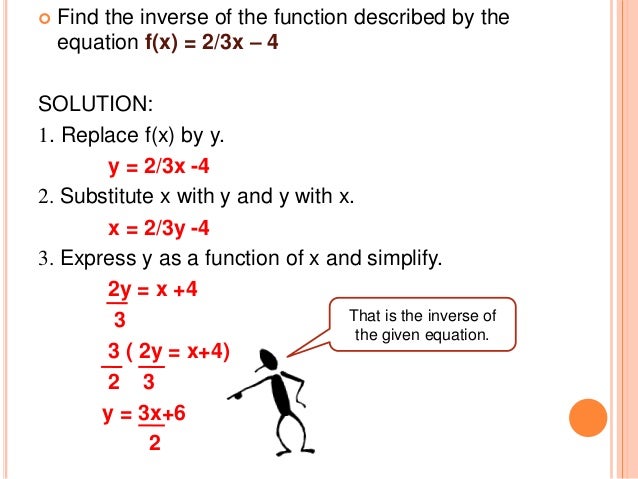





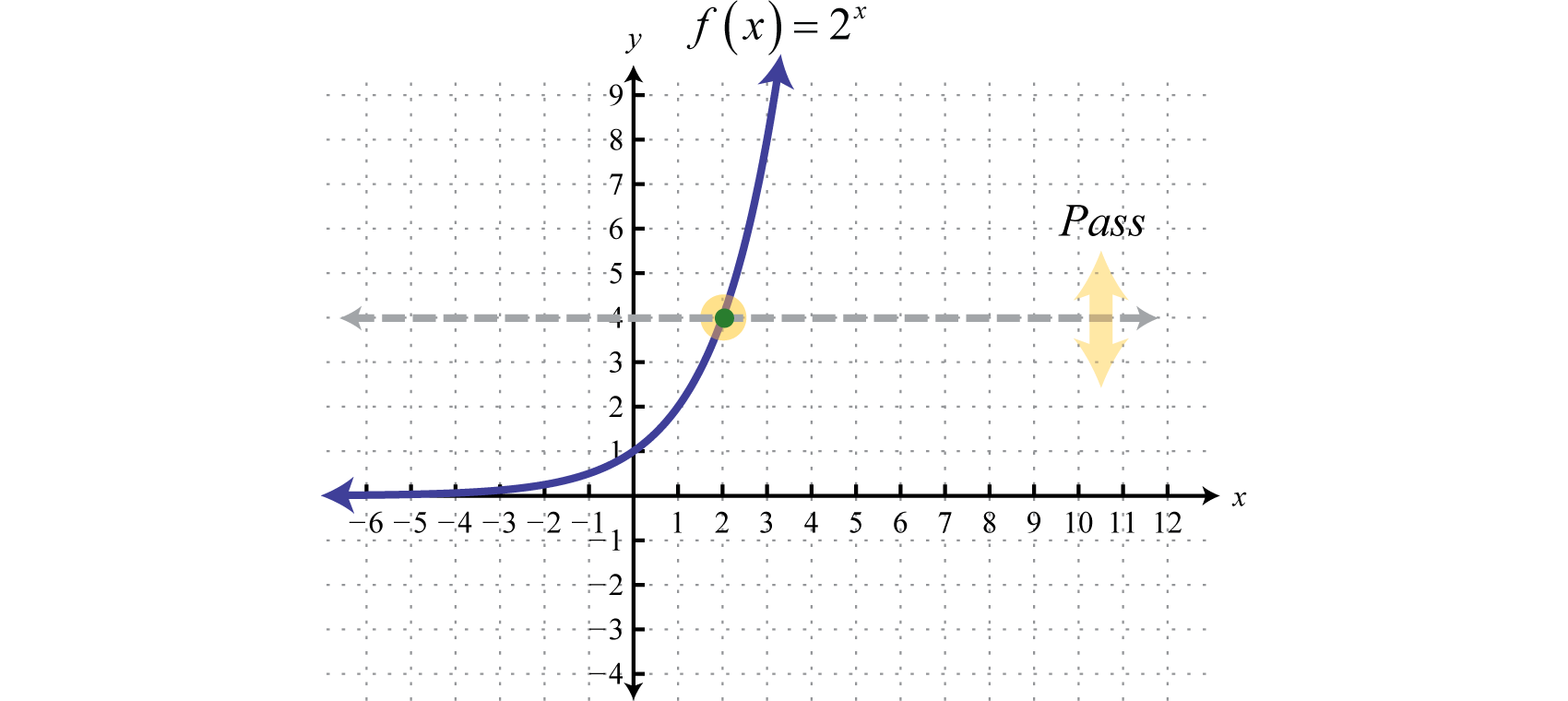


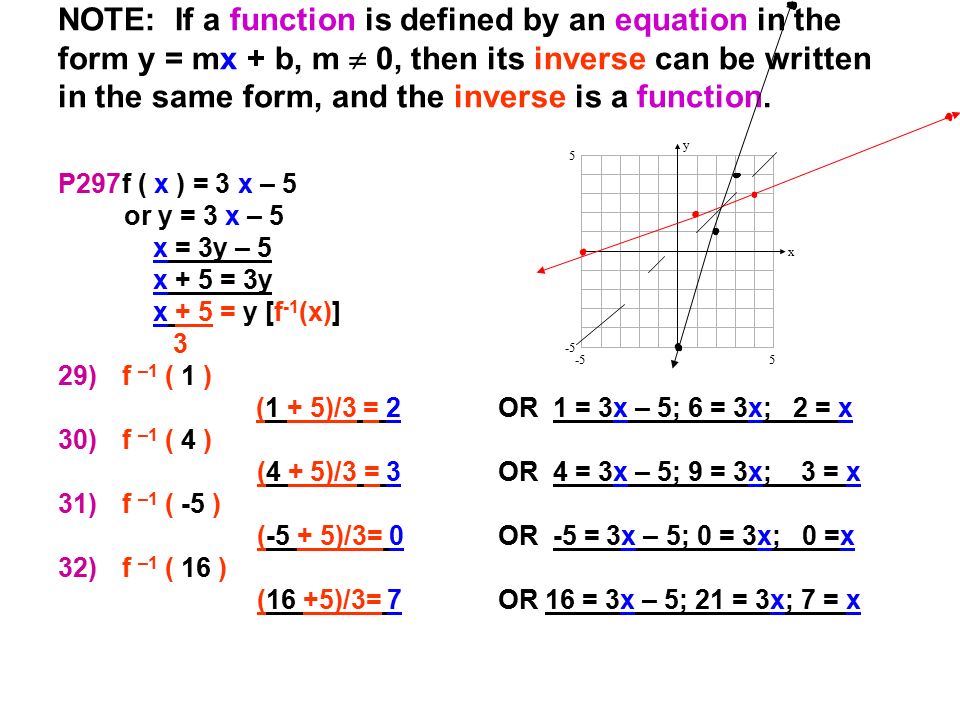

















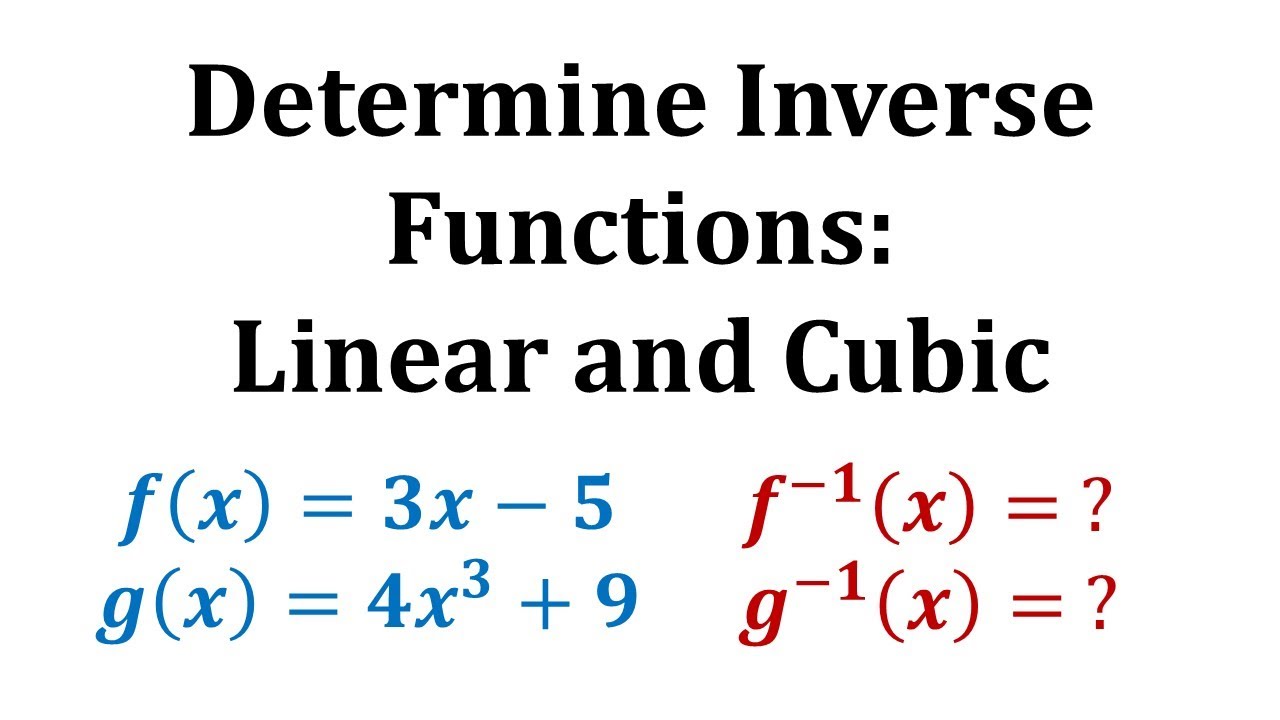










0 件のコメント:
コメントを投稿